从终点往起点倒推 .
在一个图 考虑点 u , 出度为 s : s &＃61; 0 , d[ u ] &＃61; 0 ; s ≠ 0 , 则 d( u ) &＃61; ( ∑ d( v ) ) / s ( ( u , v ) ∈ E )
----------------------------------------------------------------------------
#include
#include
#include
#include
#include
#include
#define clr( x , c ) memset( x , c , sizeof( x ) )
#define rep( i , n ) for( int i &＃61; 0 ; i
#define mp make_pair
using namespace std;
typedef pair pii;
const int maxn &＃61; 1e5 &＃43; 5;
vector g[ maxn ]; // { to , dist }
int n;
double d[ maxn ];
bool vis[ maxn ];
void dfs( int x ) {
vis[ x ] &＃61; true;
int s &＃61; g[ x ].size();
rep( i , s ) {
if( ! vis[ g[ x ][ i ].first ] ) dfs( g[ x ][ i ].first );
d[ x ] &＃43;&＃61; d[ g[ x ][ i ].first ] &＃43; g[ x ][ i ].second;
}
if( s ) d[ x ] /&＃61; s;
}
int main() {
//freopen( "test.in" , "r" , stdin );
int m;
cin >> n >> m;
while( m-- ) {
int u , v , d;
scanf( "%d%d%d" , &u , &v , &d );
--u , --v;
g[ u ].push_back( mp( v , d ) );
}
clr( d , 0 ) , clr( vis , 0 );
dfs( 0 );
printf( "%.2lf\n" , d[ 0 ] );
return 0;
}
----------------------------------------------------------------------------
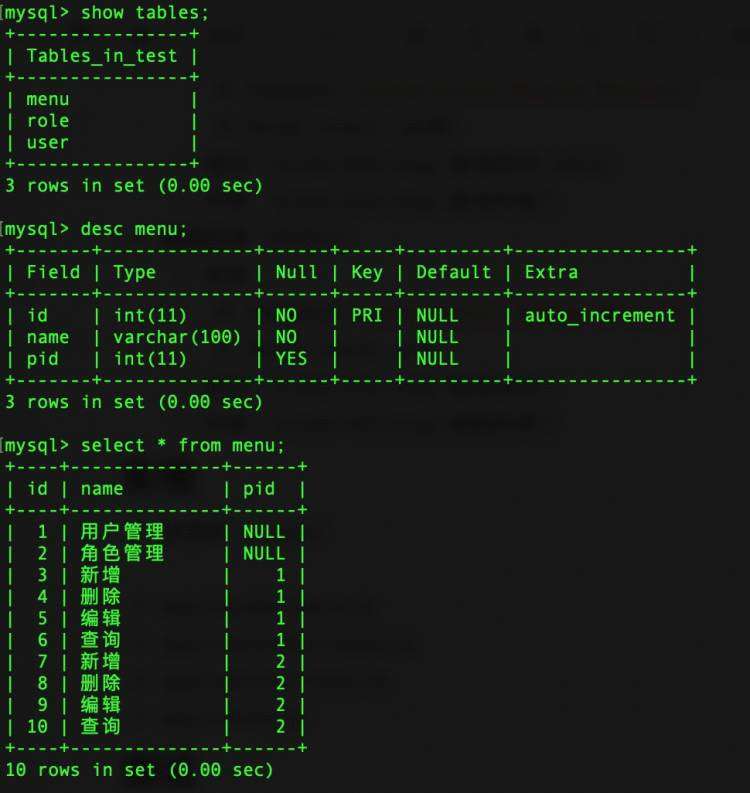
3036: 绿豆蛙的归宿
Time Limit: 2 Sec Memory Limit: 128 MBSubmit: 211 Solved: 149
[Submit][Status][Discuss]
Description
随着新版百度空间的下线&＃xff0c;Blog宠物绿豆蛙完成了它的使命&＃xff0c;去寻找它新的归宿。
给出一个有向无环的连通图&＃xff0c;起点为1终点为N&＃xff0c;每条边都有一个长度。绿豆蛙从起点出发&＃xff0c;走向终点。
到达每一个顶点时&＃xff0c;如果有K条离开该点的道路&＃xff0c;绿豆蛙可以选择任意一条道路离开该点&＃xff0c;并且走向每条路的概率为 1/K 。
现在绿豆蛙想知道&＃xff0c;从起点走到终点的所经过的路径总长度期望是多少&＃xff1f;
Input
第一行: 两个整数 N M&＃xff0c;代表图中有N个点、M条边
第二行到第 1&＃43;M 行: 每行3个整数 a b c&＃xff0c;代表从a到b有一条长度为c的有向边
Output
从起点到终点路径总长度的期望值&＃xff0c;四舍五入保留两位小数。
Sample Input
4 4
1 2 1
1 3 2
2 3 3
3 4 4
1 2 1
1 3 2
2 3 3
3 4 4
Sample Output
7.00
HINT
对于100%的数据 N<&＃61;100000&＃xff0c;M<&＃61;2*N
Source
Poetize3
京公网安备 11010802041100号